Structure-preserving regularization and stochastic forcing for nonlinear hyperbolic PDEs (SPReSto)
Organization:
Description:
Nonlinear hyperbolic systems of partial differential equations (PDEs) play an important role in many natural processes. One of these topics, which has gained an increasing amount of attention in research recently, is ocean climate modelling. The equations governing these systems often display a wide range of dynamic scales that cannot be handled in all detail with current computing resources and numerical methods. For this reason, an intuitive solution to this problem is to develop approximate, coarsened models which can provide adequate answers that do not depend much on the smallest details of the solution. Such coarsened, yet sufficiently accurate and practically feasible approximation frameworks are the central objects of investigation in this project.
The crucial step in turning general regularizations of the model into accurate prediction methodologies lies in the preservation of the dynamic structure of the model, e.g., expressed by an underlying dynamic principle such Kelvin’s circulation theorem or conservation of energy. The aim of this project is to develop structure-preserving numerical methods for these systems of PDEs.
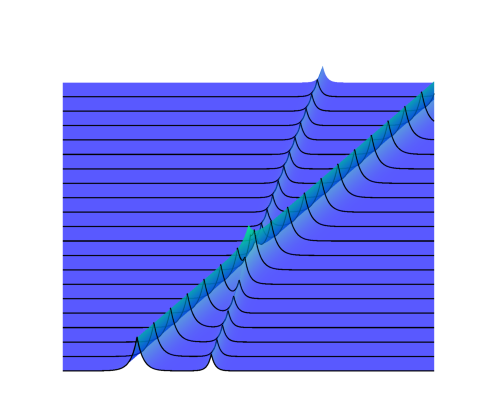
A first result based on the numerical solution of the Camassa-Holm equation is shown below, illustrating two solitons (peakons) interacting with each other. This is a basic problem for which problem-specific structure-preserving discretizations will be developed.
Output:
Publications:
Pictures: