Efficient state and parameter estimation in very large systems
Organization:
Funded by: | European Union under the Marie Curie Actions in the 7th Framework Program |
PhD: | Wenbo Wang |
Supervisor: | Pranab Mandal |
Collaboration: | TRAX project is in collaboration with 7 other European partners -- Thales Nederland, XSens (NL), Rinicom, University of Sheffield (UK), Ericsson, Linkoping University (SE), and Fraunhoffer (DE |
Description:
Statistical signal processing and, in particular, filtering of signals plays an ever-increasing role in a wide range of areas from object tracking using radar or sensors to volatility estimation in financial markets, from speech recognition to weather forecasting, from medical care to precision agriculture. The main goal is to extract information about the unobservable signals based on all the observations up to the current time and preferably, recursively in time. The sequential Monte Carlo (SMC) methods, also known as Particle Filters, became a set of powerful and versatile simulation-based tool to estimate, recursively, the conditional density of the unobserved signal from the noisy observations. The main advantage of this method is that it can very easily adapt to any possible nonlinearity in the model and/or possible non-Gaussianity of the noise processes involved. As the use of stochastic filtering theory widens in practice, we need to deal with more and more complex systems, be it because of huge amount of data containing information about the signal from different sources, or be it because of very high-dimensional state and parameters in the model. Examples include multi target tracking from the radar measurements or human motion tracking from data obtained from different sensors fixed at different body parts, which involves a lot of unknown parameters.
In this project, we shall study and exploit the specific structure present in a high-dimensional system to develop efficient techniques and better algorithms for state and parameter estimation. Proposal densities that will help with high-dimensional situation will be studied and characterized for specific system structures. The idea of ensemble particle filter will also be explored to handle large systems
Publications:
Pictures:
| Fig1, Multiple targets tracking, real-time estimation of parameter and state in a large system (such as multiple targets tracking) is still challenging. |
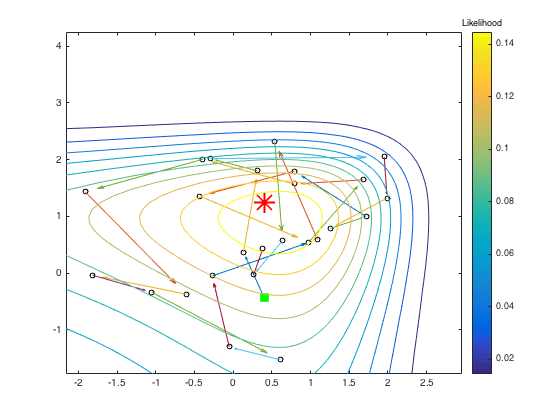
| Fig2, MCMC method (Metropolis-Hastings Random Walk) for estimation purpose. This figure shows that the trajectory of every step random walk, the different level of p(x|y) likelihood and the initial estimated point (the green filled square), the true signal (the red star). |