Organization:
Funded by: Rubicon Fellowship from NWO (Netherlands Organisation for Scientific Research).
Postdoc: Sander Rhebergen
Supervisor: Jaap van der Vegt
Collaboration: Sander Rhebergen (University of Minnesota)
Description:
Space-time discontinuous Galerkin (DG) methods combine the efficiency of dealing with deforming grids, in which the geometric conservation law is automatically satisfied, with all the benefits of standard DG methods, i.e., their ability to efficiently deal with unstructured grids, local mesh refinement (h-adaptation), adjustment of the polynomial order (p-refinement) and parallel computation. These benefits stem from the use of discontinuous basis functions in both space and time, resulting in a compact stencil of the discretization. Furthermore, (space-time) DG methods easily deal with shocks and other discontinuities in the solution.
Space-time DG discretizations of partial differential equations result in large systems of (non)linear algebraic equations for the polynomial expansions in each element. Extending the space-time discretizations to higher order accuracy is straightforward, but current solvers are inefficient in solving these discretizations.
In this project we are developing efficient solvers for higher order accurate space-time DG methods. We have developed an algorithm that combines p-multigrid with h-multigrid as smoother in the p-multigrid at all p-levels. By using semi-coarsening in combination with a new semi-implicit Runge-Kutta method as smoother, we further enhanced the performance of our hp-Multigrid as Smoother algorithm (hp-MGS). An extensive multilevel Fourier analysis of the hp-MGS algorithm was conducted to obtain more insight into the theoretical performance of the algorithm and to optimize the coefficients in the semi-implicit Runge-Kutta smoother. The analysis and numerical test cases show that excellent multigrid convergence is obtained for low and high Reynolds numbers and on highly stretched meshes.
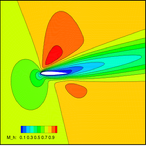
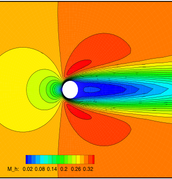
"Higher-order accurate space-time DG discretizations for compressible flow."
Publications:
Ph.D. Thesis:
S. Rhebergen, Discontinuous Galerkin finite element methods for (non)conservative partial differential equations, (2010).
